Tuesday 31 July, 2007, 21:26 - Radio Randomness
Anyone who really knows their stuff when it comes to radio propagation and the like will tell you that any aerial (or an antenna for that matter) has a certain capture area.  Its capture area defines, in effect, how much of the signal that is in the ether it can capture and thus present to an attached receiver. The bigger the aerial, the greater its capture area and the stronger the received signal. Obvious really.
Its capture area defines, in effect, how much of the signal that is in the ether it can capture and thus present to an attached receiver. The bigger the aerial, the greater its capture area and the stronger the received signal. Obvious really.For some antennas, such as satellite dishes, the capture area is relatively obvious. A satellite dish which covers an area of 1 square metre has a capture area of roughly 1 square metre (ignoring edge effects). For linear aerials, however, the capture area is less obvious. Which has the greatest gain - a 4 element yagi or a 4 wavelength long colinear? Not so easy is it. However, it seemed to me that there ought to be a simple rule-of-thumb which allowed simple comparison between antennas. So let's therefore hypothesise that:
- The gain of a linear aerial ought to be somehow related to the amount (length) of metal it represents. Thus if you add up the length of the elements on a yagi and stretch them out into a colinear, the two should have the same gain.
- A doubling of the amount (length) of metal should double the overall gain (i.e. increase it by 3dB).
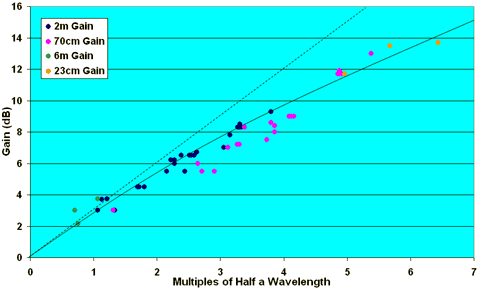
The dotted line represents the hypothesis - i.e. that the gain rises by 3dB for each doubling of length. The solid line represents a 'best fit' line. As the antenna gets long (in terms of wavelengths) there is a noticeable drop in gain with respect to the hypothesis. It is probable that as the antennas become long, the losses in the 'metal' itself cause a fall in total gain, away from the the theoretical figure. Alternatively the hypothesis is incorrect. Either way, the original premise isn't that far off!
The next job is to see whether the hypothesis about the total length of elements of a yagi and the length of 'wire' in a a colinear holds any water.
